Truncated order-6 hexagonal tiling

In today's world, interest in Truncated order-6 hexagonal tiling has been constantly increasing. Whether due to its relevance today or its impact on history, Truncated order-6 hexagonal tiling has captured the attention of a wide audience. Over the years, debates, research and studies have been generated that have delved into its meaning and significance. Both in the academic and popular spheres, Truncated order-6 hexagonal tiling has aroused genuine interest and has generated all kinds of reflections and discussions. In this article, we will delve into the world of Truncated order-6 hexagonal tiling and explore its many facets, with the goal of better understanding its importance and relevance in today's society.

Truncated order-6 hexagonal tiling
Truncated order-6 hexagonal tiling
Poincaré disk model of the hyperbolic plane
Type Hyperbolic uniform tiling
Vertex configuration 6.12.12
Schläfli symbol t{6,6} or h2{4,6}
t(6,6,3)
Wythoff symbol 2 6 | 6
3 6 6 |
Coxeter diagram =
=
Symmetry group , (*662)
, (*663)
Dual Order-6 hexakis hexagonal tiling
Properties Vertex-transitive

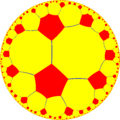
In geometry, the truncated order-6 hexagonal tiling is a uniform tiling of the hyperbolic plane. It has Schläfli symbol of t{6,6}. It can also be identically constructed as a cantic order-6 square tiling, h2{4,6}

Uniform colorings

By *663 symmetry, this tiling can be constructed as an omnitruncation, t{(6,6,3)}:

Symmetry

Truncated order-6 hexagonal tiling with *663 mirror lines

The dual to this tiling represent the fundamental domains of (*663) symmetry. There are 3 small index subgroup symmetries constructed from by mirror removal and alternation. In these images fundamental domains are alternately colored black and white, and mirrors exist on the boundaries between colors.

The symmetry can be doubled as 662 symmetry by adding a mirror bisecting the fundamental domain.

Small index subgroups of (*663)
Index 1 2 6
Diagram
Coxeter
(orbifold) 		=
(*663) 		= =
(*3333) 		=
(3*33) 		=
(*333333)
Direct subgroups
Index 2 4 12
Diagram
Coxeter
(orbifold) 		+ =
(663) 		+ = =
(3333) 		+ =
(333333)
Uniform hexahexagonal tilings
Symmetry: , (*662)
=
= 		=
= 		=
= 		=
= 		=
= 		=
= 		=
=
{6,6}
= h{4,6} 		t{6,6}
= h2{4,6} 		r{6,6}
{6,4} 		t{6,6}
= h2{4,6} 		{6,6}
= h{4,6} 		rr{6,6}
r{6,4} 		tr{6,6}
t{6,4}
Uniform duals
V66 V6.12.12 V6.6.6.6 V6.12.12 V66 V4.6.4.6 V4.12.12
Alternations

(*663)
(6*3)
(*3232)
(6*3)
(*663)
(2*33) 		+
(662)
= = =
h{6,6} s{6,6} hr{6,6} s{6,6} h{6,6} hrr{6,6} sr{6,6}

References

  • John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
  • "Chapter 10: Regular honeycombs in hyperbolic space". The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678.

See also